Hexagonal tiling honeycomb
| Hexagonal tiling honeycomb | |
|---|---|
 Perspective projection view within Poincaré disk model | |
| Type | Hyperbolic regular honeycomb Paracompact uniform honeycomb |
| Schläfli symbols | {6,3,3} t{3,6,3} 2t{6,3,6} 2t{6,3[3]} t{3[3,3]} |
| Coxeter diagrams | |
| Cells | {6,3}  |
| Faces | Hexagon {6} |
| Edge figure | Triangle {3} |
| Vertex figure | 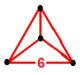 tetrahedron {3,3} |
| Dual | {3,3,6} |
| Coxeter groups | , [6,3,3] , [3,6,3] , [6,3,6] , [6,3[3]] , [3[3,3]] |
| Properties | Regular |
In the field of hyperbolic geometry, the hexagonal tiling honeycomb arises one of 11 regular paracompact honeycombs in 3-dimensional hyperbolic space. It is called paracompact because it has infinite cells. Each cell consists of a hexagonal tiling whose vertices lie on a horosphere: a flat plane in hyperbolic space that approaches a single ideal point at infinity.
The Schläfli symbol of the hexagonal tiling honeycomb is {6,3,3}. Since that of the hexagonal tiling of the plane is {6,3}, this honeycomb has three such hexagonal tilings meeting at each edge. Since the Schläfli symbol of the tetrahedron is {3,3}, the vertex figure of this honeycomb is an tetrahedron. Thus, six hexagonal tilings meet at each vertex of this honeycomb, and four edges meet at each vertex.[1]
Images

Viewed in perspective outside of a Poincaré disk model, this shows one hexagonal tiling cell within the honeycomb, and its mid-radius horosphere (the horosphere incident with edge midpoints). In this projection, the hexagons grow infinitely small towards the infinite boundary asymptoting towards a single ideal point. It can be seen as similar to the order-3 apeirogonal tiling, {∞,3} of H2, with horocycle circumscribing vertices of apeirogonal faces.
| {6,3,3} | {∞,3} |
|---|---|
 |
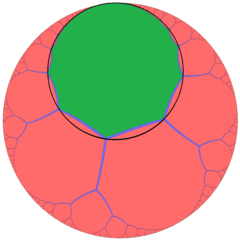 |
| One hexagonal tiling of this honeycomb | order-3 apeirogonal tiling with a green apeirogon and its horocycle |
Symmetry constructions
It has a total of five reflectional constructions from five related Coxeter groups all with four mirrors and only the first being regular: ![]()
![]()
![]()
![]()
![]()
![]()
![]() [6,3,3],
[6,3,3], ![]()
![]()
![]()
![]()
![]()
![]()
![]() [3,6,3],
[3,6,3], ![]()
![]()
![]()
![]()
![]()
![]()
![]() [6,3,6],
[6,3,6], ![]()
![]()
![]()
![]()
![]() [6,3[3]] and [3[3,3]]
[6,3[3]] and [3[3,3]] ![]()
![]()
![]() , having 1, 4, 6, 12 and 24 times larger fundamental domains respectively. In Coxeter notation subgroup markups, they are related as: [6,(3,3)*] (remove 3 mirrors, index 24 subgroup); [3,6,3*] or [3*,6,3] (remove 2 mirrors, index 6 subgroup); [1+,6,3,6,1+] (remove two orthogonal mirrors, index 4 subgroup); all of these are isomorphic to [3[3,3]]. The ringed Coxeter diagrams are
, having 1, 4, 6, 12 and 24 times larger fundamental domains respectively. In Coxeter notation subgroup markups, they are related as: [6,(3,3)*] (remove 3 mirrors, index 24 subgroup); [3,6,3*] or [3*,6,3] (remove 2 mirrors, index 6 subgroup); [1+,6,3,6,1+] (remove two orthogonal mirrors, index 4 subgroup); all of these are isomorphic to [3[3,3]]. The ringed Coxeter diagrams are ![]()
![]()
![]()
![]()
![]()
![]()
![]() ,
, ![]()
![]()
![]()
![]()
![]()
![]()
![]() ,
, ![]()
![]()
![]()
![]()
![]()
![]()
![]() ,
, ![]()
![]()
![]()
![]()
![]() and
and ![]()
![]()
![]() , representing different types (colors) of hexagonal tilings in the Wythoff construction.
, representing different types (colors) of hexagonal tilings in the Wythoff construction.
Related polytopes and honeycombs
It is one of 15 regular hyperbolic honeycombs in 3-space, 11 of which like this one are paracompact, with infinite cells or vertex figures.
| 11 paracompact regular honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
 {6,3,3} |
 {6,3,4} |
 {6,3,5} |
 {6,3,6} |
 {4,4,3} |
 {4,4,4} | ||||||
 {3,3,6} |
 {4,3,6} |
 {5,3,6} |
 {3,6,3} |
 {3,4,4} | |||||||
It is one of 15 uniform paracompact honeycombs in the [6,3,3] Coxeter group, along with its dual, the order-6 tetrahedral honeycomb, {3,3,6}.
| [6,3,3] family honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| {6,3,3} | r{6,3,3} | t{6,3,3} | rr{6,3,3} | t0,3{6,3,3} | tr{6,3,3} | t0,1,3{6,3,3} | t0,1,2,3{6,3,3} | ||||
 |
 |
 |
 |
 |
 |
 |
 | ||||
 |
 |
 |
 |
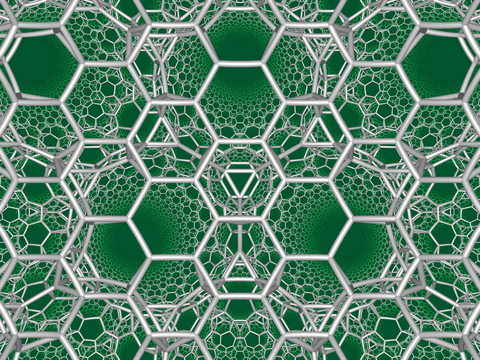 |
 |
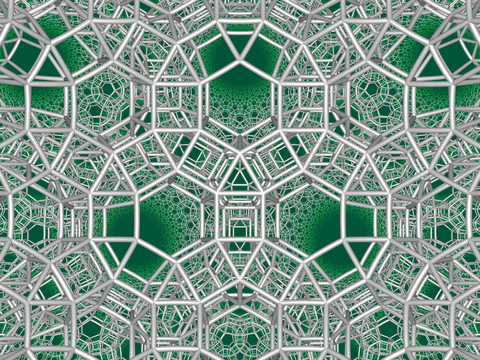 | |||||
| {3,3,6} | r{3,3,6} | t{3,3,6} | rr{3,3,6} | 2t{3,3,6} | tr{3,3,6} | t0,1,3{3,3,6} | t0,1,2,3{3,3,6} | ||||
Polytopes and honeycombs with tetrahedral vertex figures
It is in a sequence with regular polychora: 5-cell {3,3,3}, tesseract {4,3,3}, 120-cell {5,3,3} of Euclidean 4-space, with tetrahedral vertex figures.
| {p,3,3} honeycombs | ||||||||
|---|---|---|---|---|---|---|---|---|
| Space | S3 | H3 | ||||||
| Form | Finite | Paracompact | Noncompact | |||||
| Name | {3,3,3} | {4,3,3} | {5,3,3} | {6,3,3} | {7,3,3} | {8,3,3} | ... {∞,3,3} | |
| Image |  |
 |
 |
 |
 |
|||
Coxeter diagrams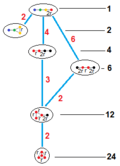 |
1 | |||||||
| 4 | ||||||||
| 6 | ||||||||
| 12 | ||||||||
| 24 | ||||||||
| Cells {p,3} |
 {3,3} |
 {4,3} |
 {5,3} |
 {6,3} |
 {7,3} |
 {8,3} |
 {∞,3} | |
Polytopes and honeycombs with hexagonal tiling cells
It is a part of sequence of regular honeycombs of the form {6,3,p}, with hexagonal tiling cells:
| {6,3,p} honeycombs | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Space | H3 | ||||||||||
| Form | Paracompact | Noncompact | |||||||||
| Name | {6,3,3} | {6,3,4} | {6,3,5} | {6,3,6} | {6,3,7} |
{6,3,8} |
... {6,3,∞} | ||||
| Coxeter |
|||||||||||
| Image |  |
 |
 |
 |
 |
 | |||||
| Vertex figure {3,p} |
{3,3} |
{3,4} |
{3,5} |
{3,6} |
{3,7} |
{3,8} |
{3,∞} | ||||
Rectified hexagonal tiling honeycomb
| Rectified hexagonal tiling honeycomb | |
|---|---|
 | |
| Type | Paracompact uniform honeycomb |
| Schläfli symbols | r{6,3,3} or t1{6,3,3} |
| Coxeter diagrams | |
| Cells | {3,3} r{6,3} |
| Faces | Triangle {3} Hexagon {6} |
| Vertex figure | 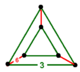 Triangular prism {}×{3} |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive, edge-transitive |
The rectified hexagonal tiling honeycomb, t1{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has tetrahedral and trihexagonal tiling facets, with a triangular prism vertex figure. The
has tetrahedral and trihexagonal tiling facets, with a triangular prism vertex figure. The ![]()
![]()
![]()
![]()
![]() half-symmetry construction alternate two types of tetrahedra.
half-symmetry construction alternate two types of tetrahedra.
| Hexagonal tiling honeycomb |
Rectified hexagonal tiling honeycomb |
|---|---|
 |
 |
| Related H2 tilings | |
| Order-3 apeirogonal tiling |
Triapeirogonal tiling |
 |
  |
Truncated hexagonal tiling honeycomb
| Truncated hexagonal tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | t{6,3,3} or t0,1{6,3,3} |
| Coxeter diagram | |
| Cells | {3,3} t{6,3} |
| Faces | Triangle {3} Dodecagon {12} |
| Vertex figure |  tetrahedron |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The truncated hexagonal tiling honeycomb, t0,1{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has tetrahedral and truncated hexagonal tiling facets, with a tetrahedral vertex figure.
has tetrahedral and truncated hexagonal tiling facets, with a tetrahedral vertex figure.

It is similar to the 2D hyperbolic truncated order-3 apeirogonal tiling, t{∞,3} with apeirogonal and triangle faces:
Bitruncated hexagonal tiling honeycomb
| Bitruncated hexagonal tiling honeycomb Bitruncated order-6 tetrahedral honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | 2t{6,3,3} or t1,2{6,3,3} |
| Coxeter diagram | |
| Cells | t{3,3} t{3,6} |
| Faces | Triangle {3} hexagon {6} |
| Vertex figure |  tetrahedron |
| Coxeter groups | , [6,3,3] , [3,3[3]] |
| Properties | Vertex-transitive |
The bitruncated hexagonal tiling honeycomb or bitruncated order-6 tetrahedral honeycomb, t1,2{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated tetrahedra and hexagonal tiling cells, with a tetrahedral vertex figure.
has truncated tetrahedra and hexagonal tiling cells, with a tetrahedral vertex figure.

Cantellated hexagonal tiling honeycomb
| Cantellated hexagonal tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | rr{6,3,3} or t0,2{6,3,3} |
| Coxeter diagram | |
| Cells | r{3,3} rr{6,3} {}×{3} |
| Faces | Triangle {3} Square {4} Hexagon {6} |
| Vertex figure | Irreg. triangular prism |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The cantellated hexagonal tiling honeycomb, t0,2{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has octahedral and rhombitrihexagonal tiling cells, with a triangular prism vertex figure.
has octahedral and rhombitrihexagonal tiling cells, with a triangular prism vertex figure.

Cantitruncated hexagonal tiling honeycomb
| Cantitruncated hexagonal tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | tr{6,3,3} or t0,1,2{6,3,3} |
| Coxeter diagram | |
| Cells | t{3,3} tr{6,3} {}×{3} |
| Faces | Triangle {3} Square {4} Hexagon {6} |
| Vertex figure |  Irreg. tetrahedron |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The cantitruncated hexagonal tiling honeycomb, t0,1,2{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated tetrahedron and truncated trihexagonal tiling cells, with a tetrahedron vertex figure.
has truncated tetrahedron and truncated trihexagonal tiling cells, with a tetrahedron vertex figure.

Runcinated hexagonal tiling honeycomb
| Runcinated hexagonal tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | t0,3{6,3,3} |
| Coxeter diagram | |
| Cells | {3,3} t0,2{6,3} {}×{6} {}×{3} |
| Faces | Triangle {3} Square {4} Hexagon {6} |
| Vertex figure |  Octahedron |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The runcinated hexagonal tiling honeycomb, t0,3{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has tetrahedron, rhombitrihexagonal tiling hexagonal prism, triangular prism cells, with a octahedron vertex figure.
has tetrahedron, rhombitrihexagonal tiling hexagonal prism, triangular prism cells, with a octahedron vertex figure.

Runcitruncated hexagonal tiling honeycomb
| Runcitruncated hexagonal tiling honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | t0,1,3{6,3,3} |
| Coxeter diagram | |
| Cells | rr{3,3} {}x{3} {}x{12} t{6,3} |
| Faces | Triangle {3} Square {4} Hexagon {6} Dodecagon {12} |
| Vertex figure | quad-pyramid |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The runcitruncated hexagonal tiling honeycomb, t0,1,3{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has cuboctahedron, Triangular prism, Dodecagonal prism, and truncated hexagonal tiling cells, with a quad-pyramid vertex figure.
has cuboctahedron, Triangular prism, Dodecagonal prism, and truncated hexagonal tiling cells, with a quad-pyramid vertex figure.

Runcicantellated hexagonal tiling honeycomb
| Runcicantellated hexagonal tiling honeycomb runcitruncated order-6 tetrahedral honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | t0,2,3{6,3,3} |
| Coxeter diagram | |
| Cells | t{3,3} {}x{6} rr{6,3} |
| Faces | Triangle {3} Square {4} Hexagon {6} |
| Vertex figure | quad-pyramid |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The runcicantellated hexagonal tiling honeycomb or runcitruncated order-6 tetrahedral honeycomb, t0,2,3{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated tetrahedron, hexagonal prism, hexagonal prism, and rhombitrihexagonal tiling cells, with a quad-pyramid vertex figure.
has truncated tetrahedron, hexagonal prism, hexagonal prism, and rhombitrihexagonal tiling cells, with a quad-pyramid vertex figure.

Omnitruncated hexagonal tiling honeycomb
| Omnitruncated hexagonal tiling honeycomb Omnitruncated order-6 tetrahedral honeycomb | |
|---|---|
| Type | Paracompact uniform honeycomb |
| Schläfli symbol | t0,1,2,3{6,3,3} |
| Coxeter diagram | |
| Cells | tr{3,3} {}x{6} {}x{12} tr{6,3} |
| Faces | Square {4} Hexagon {6} Dodecagon {12} |
| Vertex figure |  tetrahedron |
| Coxeter groups | , [6,3,3] |
| Properties | Vertex-transitive |
The omnitruncated hexagonal tiling honeycomb or omnitruncated order-6 tetrahedral honeycomb, t0,1,2,3{6,3,3}, ![]()
![]()
![]()
![]()
![]()
![]()
![]() has truncated octahedron, hexagonal prism, dodecagonal prism, and truncated trihexagonal tiling cells, with a quad-pyramid vertex figure.
has truncated octahedron, hexagonal prism, dodecagonal prism, and truncated trihexagonal tiling cells, with a quad-pyramid vertex figure.

See also
References
- ↑ Coxeter The Beauty of Geometry, 1999, Chapter 10, Table III
- Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8. (Tables I and II: Regular polytopes and honeycombs, pp. 294–296)
- The Beauty of Geometry: Twelve Essays (1999), Dover Publications, LCCN 99-35678, ISBN 0-486-40919-8 (Chapter 10, Regular Honeycombs in Hyperbolic Space) Table III
- Jeffrey R. Weeks The Shape of Space, 2nd edition ISBN 0-8247-0709-5 (Chapters 16–17: Geometries on Three-manifolds I,II)
- N. W. Johnson, R. Kellerhals, J. G. Ratcliffe, S. T. Tschantz, The size of a hyperbolic Coxeter simplex, Transformation Groups (1999), Volume 4, Issue 4, pp 329–353
- N. W. Johnson, R. Kellerhals, J. G. Ratcliffe, S. T. Tschantz, Commensurability classes of hyperbolic Coxeter groups, (2002) H3: p130.
External Links
- John Baez, Visual Insight: {6,3,3} Honeycomb (2014/03/15)
- John Baez, Visual Insight: {6,3,3} Honeycomb in Upper Half Space (2013/09/15)

