Arcsine distribution

Arcsine
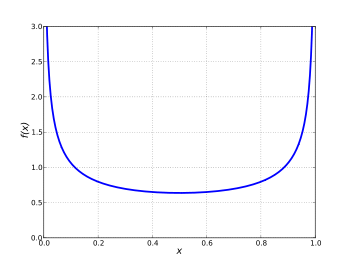
Probability density function
Cumulative distribution function
Parameters	none
Support	$x \in [0,1]$
PDF	$f(x)={\frac {1}{\pi {\sqrt {x(1-x)}}}}$
CDF	$F(x)={\frac {2}{\pi }}\arcsin \left({\sqrt x}\right)$
Mean	${\frac {1}{2}}$
Median	${\frac {1}{2}}$
Mode	$x\in \{0,1\}$
Variance	${\tfrac {1}{8}}$
Skewness	$0$
Ex. kurtosis	$-{\tfrac {3}{2}}$
Entropy	$\ln {\tfrac {\pi }{4}}$
MGF	$1+\sum _{{k=1}}^{{\infty }}\left(\prod _{{r=0}}^{{k-1}}{\frac {2r+1}{2r+2}}\right){\frac {t^{k}}{k!}}$
CF	${}_{1}F_{1}({\tfrac {1}{2}};1;i\,t)\$

In probability theory, the arcsine distribution is the probability distribution whose cumulative distribution function is

F(x)={\frac {2}{\pi }}\arcsin \left({\sqrt x}\right)={\frac {\arcsin(2x-1)}{\pi }}+{\frac {1}{2}}

for 0 ≤ x ≤ 1, and whose probability density function is

f(x)={\frac {1}{\pi {\sqrt {x(1-x)}}}}

on (0, 1). The standard arcsine distribution is a special case of the beta distribution with α = β = 1/2. That is, if $X$ is the standard arcsine distribution then $X\sim {{\rm {Beta}}}({\tfrac {1}{2}},{\tfrac {1}{2}})\$

The arcsine distribution appears

in the Lévy arcsine law;
in the Erdős arcsine law;
as the Jeffreys prior for the probability of success of a Bernoulli trial.

Generalization

Arcsine – bounded support
Parameters	$-\infty <a<b<\infty \,$
Support	$x\in [a,b]$
PDF	$f(x)={\frac {1}{\pi {\sqrt {(x-a)(b-x)}}}}$
CDF	$F(x)={\frac {2}{\pi }}\arcsin \left({\sqrt {\frac {x-a}{b-a}}}\right)$
Mean	${\frac {a+b}{2}}$
Median	${\frac {a+b}{2}}$
Mode	$x\in {a,b}$
Variance	${\tfrac {1}{8}}(b-a)^{2}$
Skewness	$0$
Ex. kurtosis	$-{\tfrac {3}{2}}$

Arbitrary bounded support

The distribution can be expanded to include any bounded support from a ≤ x ≤ b by a simple transformation

F(x)={\frac {2}{\pi }}\arcsin \left({\sqrt {\frac {x-a}{b-a}}}\right)

for a ≤ x ≤ b, and whose probability density function is

f(x)={\frac {1}{\pi {\sqrt {(x-a)(b-x)}}}}

on (a, b).

Shape factor

The generalized standard arcsine distribution on (0,1) with probability density function

f(x;\alpha )={\frac {\sin \pi \alpha }{\pi }}x^{{-\alpha }}(1-x)^{{\alpha -1}}

is also a special case of the beta distribution with parameters ${{\rm {Beta}}}(1-\alpha ,\alpha )$ .

Note that when $\alpha ={\tfrac {1}{2}}$ the general arcsine distribution reduces to the standard distribution listed above.

Properties

Arcsine distribution is closed under translation and scaling by a positive factor
- If $X\sim {{\rm {Arcsine}}}(a,b)\ {\text{then }}kX+c\sim {{\rm {Arcsine}}}(ak+c,bk+c)$
The square of an arc sine distribution over (-1, 1) has arc sine distribution over (0, 1)
- If $X\sim {{\rm {Arcsine}}}(-1,1)\ {\text{then }}X^{2}\sim {{\rm {Arcsine}}}(0,1)$

Differential equation

$\left\{2(x-1)xf'(x)+(2x-1)f(x)=0\right\}$

Related distributions

If U and V are i.i.d uniform (−π,π) random variables, then $\sin(U)$ , $\sin(2U)$ , $-\cos(2U)$ , $\sin(U+V)$ and $\sin(U-V)$ all have an ${{\rm {Arcsine}}}(-1,1)$ distribution.
If $X$ is the generalized arcsine distribution with shape parameter $\alpha$ supported on the finite interval [a,b] then ${\frac {X-a}{b-a}}\sim {{\rm {Beta}}}(1-\alpha ,\alpha )\$

References

Rogozin, B.A. (2001), "A/a013160", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4

Probability distributions

List

Discrete univariate with finite support	Benford Bernoulli beta-binomial binomial categorical hypergeometric Poisson binomial Rademacher discrete uniform Zipf Zipf–Mandelbrot

Discrete univariate with infinite support	beta negative binomial Borel Conway–Maxwell–Poisson discrete phase-type Delaporte extended negative binomial Gauss–Kuzmin geometric logarithmic negative binomial parabolic fractal Poisson Skellam Yule–Simon zeta

Continuous univariate supported on a bounded interval	arcsine ARGUS Balding–Nichols Bates beta beta rectangular Irwin–Hall Kumaraswamy logit-normal noncentral beta raised cosine reciprocal triangular U-quadratic uniform Wigner semicircle

Continuous univariate supported on a semi-infinite interval	Benini Benktander 1st kind Benktander 2nd kind beta prime Burr chi-squared chi Dagum Davis exponential-logarithmic Erlang exponential F folded normal Flory–Schulz Fréchet gamma gamma/Gompertz generalized inverse Gaussian Gompertz half-logistic half-normal Hotelling's T-squared hyper-Erlang hyperexponential hypoexponential inverse chi-squared scaled inverse chi-squared inverse Gaussian inverse gamma Kolmogorov Lévy log-Cauchy log-Laplace log-logistic log-normal matrix-exponential Maxwell–Boltzmann Maxwell–Jüttner Mittag-Leffler Nakagami noncentral chi-squared Pareto phase-type poly-Weibull Rayleigh relativistic Breit–Wigner Rice shifted Gompertz truncated normal type-2 Gumbel Weibull Discrete Weibull Wilks's lambda

Continuous univariate supported on the whole real line	Cauchy exponential power Fisher's z Gaussian q generalized normal generalized hyperbolic geometric stable Gumbel Holtsmark hyperbolic secant Johnson's S_U Landau Laplace asymmetric Laplace logistic noncentral t normal (Gaussian) normal-inverse Gaussian skew normal slash stable Student's t type-1 Gumbel Tracy–Widom variance-gamma Voigt

Continuous univariate with support whose type varies	generalized extreme value generalized Pareto Tukey lambda q-Gaussian q-exponential q-Weibull shifted log-logistic

Mixed continuous-discrete univariate	rectified Gaussian

Multivariate (joint)	Discrete Ewens multinomial Dirichlet-multinomial negative multinomial Continuous Dirichlet generalized Dirichlet multivariate normal multivariate stable multivariate t normal-inverse-gamma normal-gamma Matrix-valued inverse matrix gamma inverse-Wishart matrix normal matrix t matrix gamma normal-inverse-Wishart normal-Wishart Wishart

Directional	Univariate (circular) directional Circular uniform univariate von Mises wrapped normal wrapped Cauchy wrapped exponential wrapped asymmetric Laplace wrapped Lévy Bivariate (spherical) Kent Bivariate (toroidal) bivariate von Mises Multivariate von Mises–Fisher Bingham

Degenerate and singular	Degenerate Dirac delta function Singular Cantor

Families	Circular compound Poisson elliptical exponential natural exponential location-scale maximum entropy mixture Pearson Tweedie wrapped

Some common univariate probability distributions

Continuous	beta Cauchy chi-squared exponential F gamma Laplace log-normal normal Pareto Student's t uniform Weibull

Discrete	Bernoulli binomial discrete uniform geometric hypergeometric negative binomial Poisson

List of probability distributions

This article is issued from Wikipedia - version of the 2/22/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.