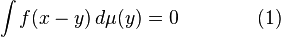Mean-periodic function

In mathematical analysis, the concept of a mean-periodic function is a generalization introduced by Jean Delsarte, of the concept of a periodic function.

Consider a complex-valued function ƒ of a real variable. The function ƒ is periodic with period a precisely if for all real x, we have ƒ(x) − ƒ(x − a) = 0. This can be written as

\int f(x-y) \, d\mu(y) = 0\qquad\qquad(1)

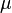where $\mu$ is the difference between the Dirac measures at 0 and a. A mean-periodic function is a function ƒ satisfying (1) for some nonzero measure 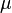$\mu$ with compact (hence bounded) support.

External links

Lectures on Mean Periodic Functions, by J. P. Kahane

This article is issued from Wikipedia - version of the 11/11/2012. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.