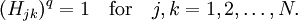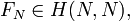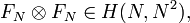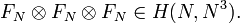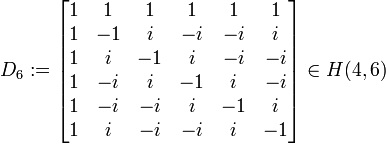Butson-type Hadamard matrix
In mathematics, a complex Hadamard matrix H of size N with all its columns (rows) mutually orthogonal, belongs to the Butson-type H(q, N) if all its elements are powers of q-th root of unity,
Existence
If p is prime then 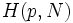 can exist
only for
can exist
only for 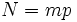 with integer m and
it is conjectured they exist for all such cases
with
with integer m and
it is conjectured they exist for all such cases
with 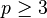 .
In general, the problem of finding all sets
.
In general, the problem of finding all sets
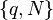 such that the Butson - type matrices
such that the Butson - type matrices
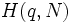 exist, remains open.
exist, remains open.
Examples
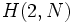 contains real Hadamard matrices of size N,
contains real Hadamard matrices of size N,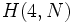 contains Hadamard matrices composed of
contains Hadamard matrices composed of 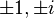 - such matrices were called by Turyn, complex Hadamard matrices.
- such matrices were called by Turyn, complex Hadamard matrices.- in the limit
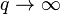 one can approximate all complex Hadamard matrices.
one can approximate all complex Hadamard matrices. - Fourier matrices
![[F_N]_{jk}:= \exp[(2\pi i(j - 1)(k - 1) / N]
{\quad \rm for \quad} j,k=1,2,\dots,N](../I/m/a2ba51c6494d8f9b4f8fd38ea5ece4b8.png)
belong to the Butson-type,
- while
-
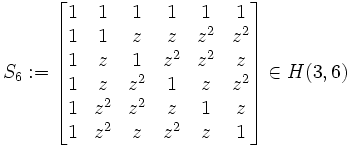 , where
, where 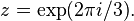
-
References
- A. T. Butson, Generalized Hadamard matrices, Proc. Am. Math. Soc. 13, 894-898 (1962).
- A. T. Butson, Relations among generalized Hadamard matrices, relative difference sets, and maximal length linear recurring sequences, Canad. J. Math. 15, 42-48 (1963).
- R. J. Turyn, Complex Hadamard matrices, pp. 435–437 in Combinatorial Structures and their Applications, Gordon and Breach, London (1970).
External links
- Complex Hadamard Matrices of Butson type - a catalogue, by Wojciech Bruzda, Wojciech Tadej and Karol Życzkowski, retrieved October 24, 2006
This article is issued from Wikipedia - version of the 3/21/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.